Heat of Fusion for Ice

Melting and freezing behavior are among the characteristic properties that give a pure substance its unique identity. As energy is added, pure solid water (ice) at 0°C changes to liquid water at 0°C.

In this experiment, you will determine the energy (in joules) required to melt one gram of ice. You will then determine the molar heat of fusion for ice (in kJ/mol). Excess ice will be added to warm water, at a known temperature, in a Styrofoam cup. The warm water will be cooled down to a temperature near 0°C by the ice. The energy required to melt the ice is removed from the warm water as it cools.

To calculate the heat that flows from the water, you can use the relationship

q = Cp•m•∆t

where q stands for heat flow, Cp is specific heat capacity, m is mass in grams, and ∆t is the change in temperature. For water, Cp is 4.18 J/g°C.

OBJECTIVES

In this experiment, you will

• Determine the energy (in Joules) required to melt one gram of ice.

• Determine the molar heat of fusion for ice (in kJ/mol).

MATERIALS

PROCEDURE

Part I Freezing

1. Connect the Temperature Probe to LabQuest and choose New from the File menu. If you have an older sensor that does not auto-ID, manually set up the sensor.

2. Use a utility clamp to clamp the Temperature Probe on a ring stand as shown in Figure 1.

3. Place a Styrofoam cup into a 250 mL beaker as shown in Figure 1.

4. Use a 100 mL graduated cylinder to obtain 100.0 mL of water at about 60°C from your teacher.

5. Obtain 7 or 8 large ice cubes.

6. On the Meter screen, tap Rate. Change the data-collection rate to 1 sample/second and the data-collection length to 480 seconds. Data collection will last 8 minutes. Select OK.

7. Lower the Temperature Probe into the warm water (to about 1 cm from the bottom).

8. Start data collection. The temperature reading, in °C, is displayed to the right of the graph. Wait until the temperature reaches a maximum (it will take a few seconds for the cold probe to reach the temperature of the warm water). This maximum will determine the initial temperature, t₁, of the water. As soon as this maximum temperature is reached, fill the Styrofoam cup with ice cubes. Shake excess water from the ice cubes before adding them (or dry with a paper towel). Record the maximum temperature, t₁, in your data table.

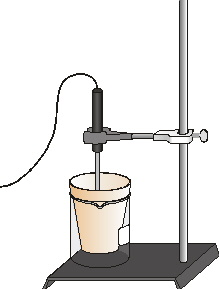
Figure 1

9. Use a stirring rod to stir the mixture as the temperature approaches 0°C. Important: As the ice melts, add more large ice cubes to keep the mixture full of ice!

10. When the temperature reaches about 4°C, quickly remove the unmelted ice (using tongs). Continue stirring until the temperature reaches a minimum (and begins to rise). This minimum temperature is the final temperature, t₂, of the water. Record t₂ in your data table.

11. Data collection will stop after 8 minutes (or stop before 8 minutes has elapsed). Use the 100 mL graduated cylinder to measure the volume of water remaining in the Styrofoam cup to the nearest 0.1 mL. Record this as V₂.

12. To confirm the t₁ and t₂ values you recorded earlier, examine the data points along the curve on the displayed graph by tapping any data point. Determine the maximum temperature, t₁, and the minimum temperature, t₂ (round to the nearest 0.1°C).

Processing the data

1. Use the equation ∆t = t₁ – t₂ to determine 916;t, the change in water temperature.

2. Subtract to determine the volume of ice that was melted (V₂ –V₁).

3. Find the mass of ice melted using the volume of melt (use 1.00 g/mL as the density of water).

4. Use the equation given in the introduction of this experiment to calculate the energy (in joules) released by the 100 g of liquid water as it cooled through ∆t.

5. Now use the results obtained above to determine the heat of fusion, the energy required to melt one gram of ice (in J/g H₂O).

6. Use your answer to Step 5 and the molar mass of water to calculate the molar heat of fusion for ice (in kJ/mol H₂O).

7. Find the percent error for the molar heat of fusion value in Step 6. The accepted value for molar heat of fusion is 6.01 kJ/mol.

DATA and calculations